VI. Using Mathematical Representations to Estimate an Interesting Quantity

Pinhole phenomena can provide a way to estimate a quantity that one can not directly measure.

A. Using pinhole phenomena to estimate the diameter of the Sun

Question 1.16 How can you use pinhole phenomena to estimate the diameter of the Sun?

How big do you think the Sun is?  It would not be possible to travel to the Sun and measure its diameter directly. Using pinhole phenomena, however, provides a way to estimate this quantity from Earth.

• In the Before section of a notebook page, record your initial estimate of the size of the Sun.

It looks about the same size as the Moon in the sky. Both seem small compared to the size of the Earth from which we are viewing them. However both are far away and things far away look smaller than they are.  How big do you think the Sun’s diameter might be?

• How could you use pinhole phenomena here on Earth to estimate the Sun’s diameter?
• A good way to start designing an exploration is to draw something that will help you think about what to do. For example, draw a ray diagram representing pinhole phenomena.
  • Which line on your ray diagram could represent the diameter of the Sun?
  • Which line could represent a projection of the Sun here on Earth?
• What equipment would you need to make such a projection of the Sun?
• How would you use this equipment?

Figure 1.21 shows one approach.

• In the During section of your physics notebook page, record what you do to measure the projection of the Sun by using such equipment.

Also draw a ray diagram to represent this setup and identify on the diagram each relevant quantity with a word or phrase and symbol.

In addition, state and justify an algebraic equation that represents the mathematical relationship among these quantities. Solve that equation for the diameter of the Sun.

Also create a table, Table I.3, that organizes the relevant information such as the name of each quantity identified, its symbol, its numerical value, and status as measured, provided, chosen, or calculated.

• In the After section of your physics notebook page, record the claim you are making about the diameter of the Sun, the evidence that you have used to support that claim, and the rationale that explains how you have interpreted the measurement you made to calculate an estimate of the Sun’s diameter.

• In the Reflection section of your physics notebook page, comment upon your experiences in using mathematical representations of pinhole phenomena to estimate an interesting quantity in this way.

What have you learned not only about the Sun but also about the scientific process of designing explorations, using geometrical reasoning, writing and justifying algebraic equations, estimating quantities, and considering whether the estimate is reasonable?

• Also record on your physics notebook page, what you are still wondering.

Complete your entry on your physics notebook page and write a summary of what you have learned before reading an example of student work in estimating the diameter of the Sun. Also read about nuances in using mathematical representations of pinhole phenomena. In addition, you may find interesting reflections about using pinhole phenomena to estimate the Sun’s diameter with friends and/or family members and some thoughts about the nature of science in this context.

1. Example of student work in estimating the diameter of the Sun

A student described the exploration process as follows:

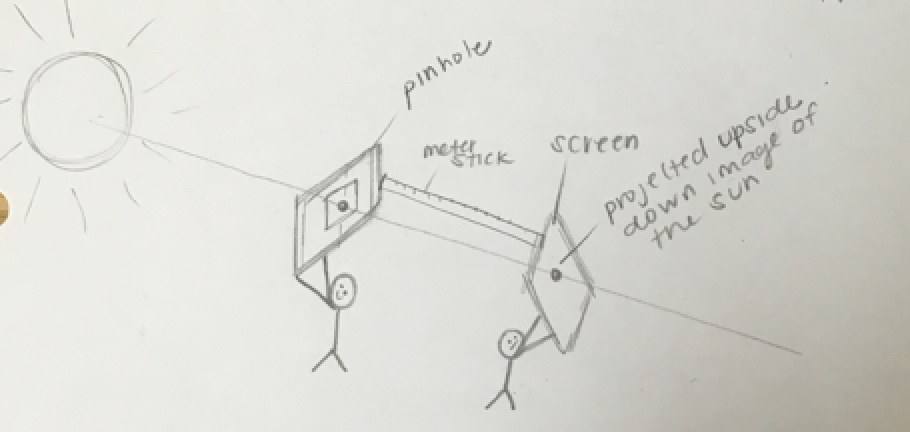
First create a camera using foil, a piece of paper, cardboard, a push pin, a meter stick, and a sunny day. First cut a square out of the middle of the cardboard and cover that opening with the foil. Poke a hole in the middle of the foil with a push pin.

To carry out the experiment, hold the screen up so that it faces the sun. Another person will hold the foil and cardboard between the sun and the screen and direct the sun rays that are traveling through the pinhole to project the upside-down image of the sun onto the screen. A third person will hold a meter stick with one end touching the screen and one end touching the cardboard with foil. A fourth person will trace the projected upside-down image of the sun that is on the paper.

Physics student, Spring 2016

The student sketched this exploration process as shown in Fig. 1.22. In this class, we referred to the diameter of the sun as its Width, represented by the symbol capital W, in order to avoid confusion with the symbol D representing the distance from the sun to the pinhole. We referred to the diameter of the Sun’s projection as its width, represented by the lower-case symbol w in order to avoid confusion with the symbol d representing the distance of the screen from the pinhole. Note that in this activity the Sun’s rays should be shown as bouncing off the opaque screen rather than shining through as in the previous pinhole activity shown in Fig. 1.15.

This student described her group’s interpretative process as follows:

The group will measure the diameter of the traced projection onto the screen. This was estimated to be about one centimeter (1 cm). This will be variable w which represents the width of the projection.

We know that the distance from the pinhole to the projection is one-meter-long (1 m) since we used a meter stick to separate the two. We will convert the one meter (1 m) to one hundred centimeters (100cm) so that the units are overall easier to work with. We will label this distance as variable d.

We are given the distance from the sun to the earth and our camera, which is about one-hundred million miles (100,000,000 mi) and will label this as variable D.

We want to find out the width of the sun so we will label this as variable W.

Since we know that this experiment creates triangles that are similar, we can set up a proportion using our known and unknown variables to determine the width of the sun.

Physics Student, Spring 2016

This student drew a ray diagram and wrote the relevant mathematics as shown in Fig. 1.23.

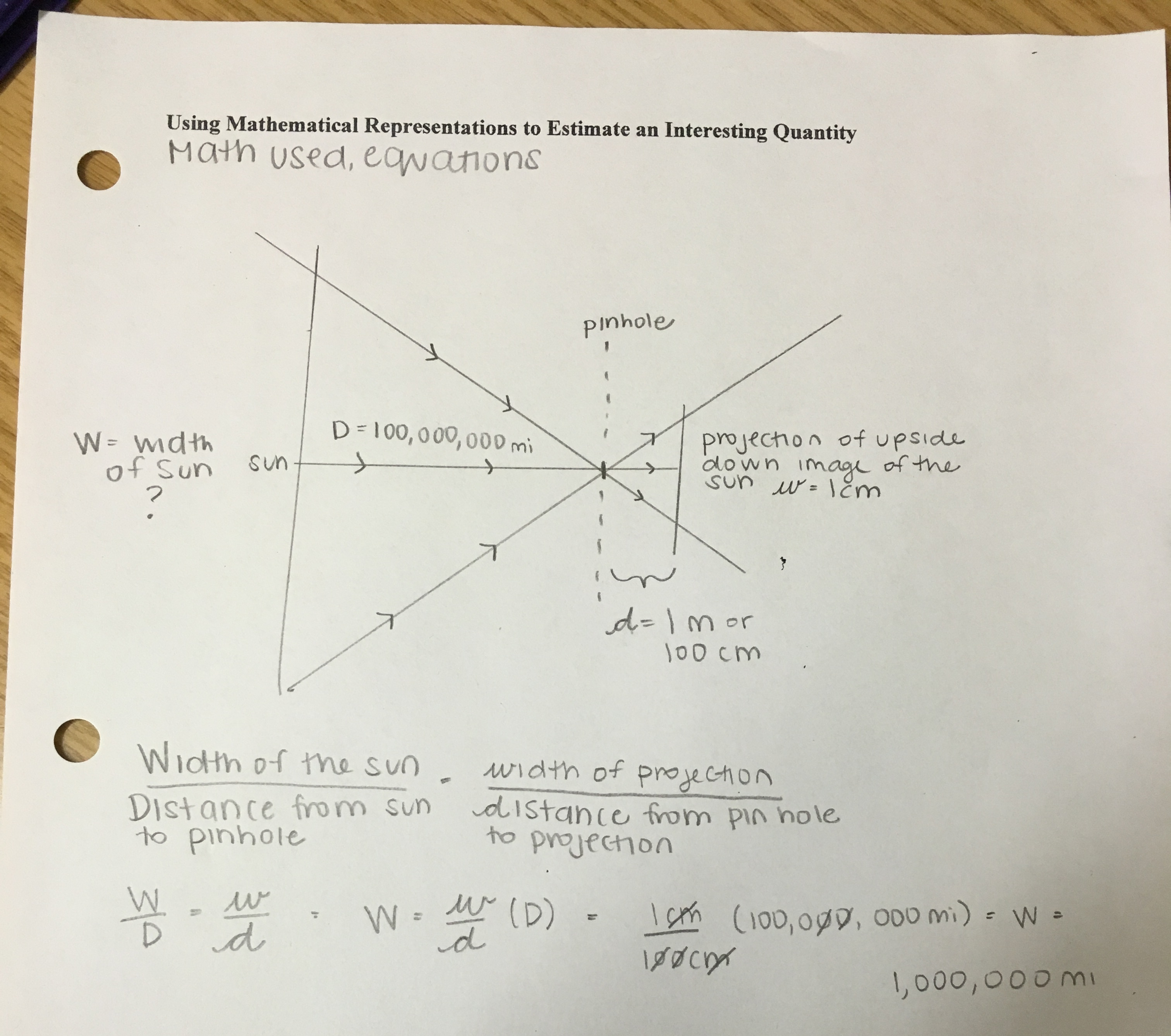
The student labeled the diagram well:

• the long vertical line on the left as representing the Sun, with the symbol W defined as the width of the Sun, and ? to indicate that this was the unknown
• the horizontal line from the “Sun” to the pinhole as the distance D, equal to about 100,000,000 miles
• the dotted line representing the foil with a pinhole in the middle as the pinhole
• the short horizontal line from the pinhole to the projection as d, equal to 1 meter or 100 centimeters
• the short vertical line on the right as representing the projection of upside down image of the Sun, with the symbol lower case w, equal to 1 centimeter.

Fig. 1.23 clearly shows the representation of pinhole phenomena by this student in estimating the diameter of the Sun. There are, however, several minor issues to consider in this otherwise excellent ray diagram. An open circle, rather than a solid line, would better represent the pinhole through which light rays are envisioned moving. A solid rather than dotted line would better represent a solid surface that blocks other rays from traveling through the pinhole.  The rays from the Sun bounced off the opaque screen back to the eyes as shown in Fig. 1.19 rather than continuing through a translucent wax paper screen as in Fig. 1.14.

This student wrote out the relevant ratios in words first before representing them with symbols:

[latex]\begin{split} \frac{\text{Width of the sun}}{\text{Distance from Sun to pinhole}} & = \frac{\text{width of projection}}{\text{distance from pinhole to projection}}\\\frac{W}{D}& = \frac{w}{d}\\W&=(\frac{w}{d})D \end{split}[/latex]

Writing out an equation in words as well as in symbols is important to do when teaching in order to be sure that students are following the reasoning.

The justification for the equal sign was that the triangle formed by the rays from the Sun and the pinhole is similar to the triangle formed by the pinhole and rays from the Sun inside the camera, and that the ratios of corresponding lengths of similar triangles are equal.

The student solved for the unknown, W = (w/d) (D),

substituted numerical values, [latex]W = \frac{1 \, cm}{100 \, cm}(100,000,000 \, miles)[/latex]

and calculated the estimate for the sun’s diameter, 1,000,000 miles.

In this case, the students did not need to convert miles to centimeters because the ratio w/d was dimensionless, 1 cm/100 cm = 1/100.

The distance of the sun to the pinhole was given as 100,000,000 miles (rather than about 93,000,000 miles) so that calculating the estimate would be easy and the attention would be on the magnitude of this estimate rather than on details involving a more accurate value for the distance to the sun. What is one hundredth of a hundred million miles?  One million miles! If you can envision what the equation is representing, one can calculate this estimate in one’s head!

The student also reported the status of the variables, as shown in Fig. 1.24 below.

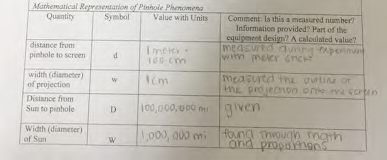
In designing and carrying out a mathematical estimation, it is useful to consider what each symbol represents. The value represented by the symbol d, for example, the distance of the projection from the pinhole, was chosen as part of the design of the exploration by the availability of a convenient tool, a meter stick of length 100 centimeters.

The diameter of the projection, w, 1 centimeter, was measured during the exploration. Some students’ tracing of the projection had a diameter of 0.9 cm; others 1.1 cm; many 1.0 cm. Using 1.0 cm seems a reasonable value given the variation in ways small groups held the pinhole and screen a meter stick length apart.

The distance D of the Sun from the Earth was an approximate value provided by the instructor based on an accepted value of about 93 million miles. This approximation made calculating the estimate easy so the students’ focus would be on the phenomena and not on detailed calculations.

The diameter of the Sun, W, was the unknown, estimated by calculating its value in the equation based upon the mathematics describing pinhole phenomena.

Is a diameter of one million miles a sensible result for an estimate of the diameter of the Sun?

What sense-making strategies can you use to consider the reasonableness of this result?

2. Some nuances in using mathematical representations of pinhole phenomena

Students often use an equal sign to represent “the next step is” to solve for the unknown variable. That is best represented by an empty space between two equations as the ratio w/d does not equal W.

After writing the equal ratios in words and the equivalent mathematical statement in symbols, this student appropriately solved for the unknown, W, with W = (w/d) (D) before substituting numerical values. This is a process that is very important to do. Substituting numerical values before solving for the unknown often leads to calculation errors.

By solving an equation for the unknown before substituting numerical values, one can readily check whether the dimensions of both sides match:

If (W in length units) = (w in length units/d in length units) (D in length units), the w/d will be dimensionless and the result will be length units on the left and length units on the right.

By solving an equation for the unknown before substituting numerical values, one also can consider whether the mathematics processes indicated are conceptually appropriate:

W = (w/d) D indicates that first one is finding a ratio of two quantities (w/d) and using that to find out how much of the distance D in the other triangle, the other width W would be.  If the little width w is half the length of the little distance d for one triangle, for example, then the big width W would be one half of the big distance D. Such visualizing of what the equation is doing is a useful way to check whether the equation one has derived makes sense.

This form of the equation, W = (w/d) (D), also makes clear that the ratio w/d is dimensionless (w/d = 1 centimeter/100 centimeters = 1/100) so there is no need to convert between centimeters and miles when substituting values. This is an example of a detail that can consume a lot of time, if one just does what one thinks one is supposed to do, converting centimeters to miles, without monitoring whether it makes sense to do so. It was necessary to convert 1 meter to 100 centimeters to use centimeters for both quantities in the ratio of w/d in order to obtain the dimensionless ratio of 1/100 but it was not necessary to convert centimeters to miles.

We used the approximate value of 100,000,000 miles for the distance between the Earth and the Sun (the distance D of the source from the pinhole). This made the calculation easy, as 1/100 of 100 million miles is 1 million miles.  Although the Sun looks small in the sky, its diameter is very large!

A “not to scale” note would be helpful, given that rays of light from the Sun are coming from so far away that they are nearly parallel as they reach the earth rather than coming in at such a large angle. Such a “not drawn to scale” also would be appropriate given that the 1 cm projection is shown as larger than the 100 centimeter distance to the pinhole. A more realistic ray diagram for this situation would be similar to Fig. 1.25:

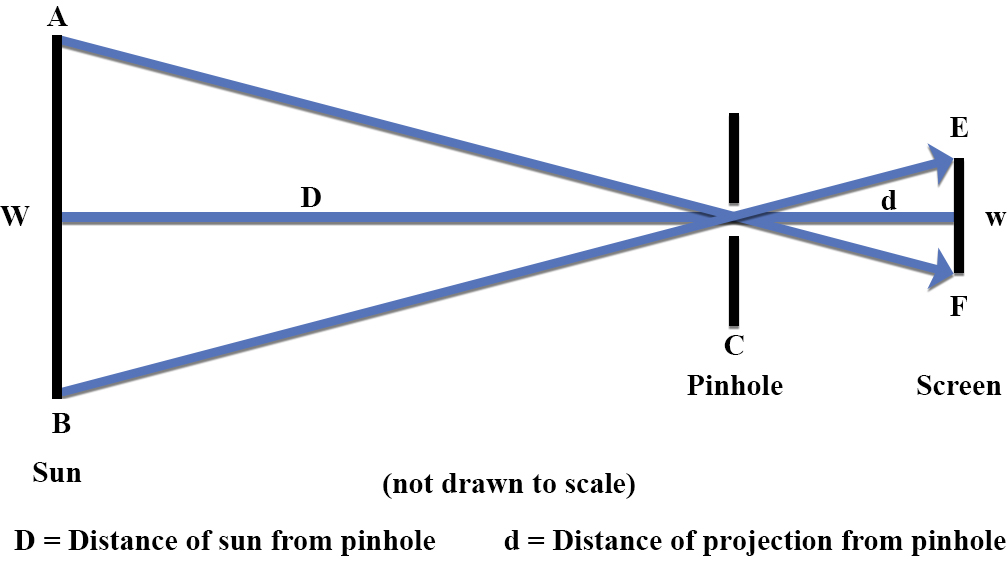
You can use your knowledge of pinhole phenomena to generate and solve pinhole math problems. To make up such a problem, decide on a scenario and specify three of the four variables involved: height of the object, H; its distance to the pinhole, D; height of its projection on the screen, h; and its distance to the pinhole, d.

In this course, the goal in solving a pinhole math problem is not the “answer.” The goal is to build your ability to help  someone else understand what to do and why. Start by helping the learner to understand what is happening by describing the scenario verbally with words and visually with a sketch. Next review the physics involved by stating what the relevant central ideas are. Also draw a careful ray diagram and use it and the central ideas to explain why the projection is upside down. Then describe the ray diagram mathematically, being clear about what each symbol represents, why the two triangles are similar, and how the lengths of similar triangles are related. Finally write the equation in both words and symbols, solve for the unknown in symbols, substitute values and calculate the answer. Be sure to also discuss why that answer seems reasonable. In facilitating a conversation with someone about pinhole phenomena, ask questions rather than tell answers throughout this process. In responding to homework problems in this course, however, follow the format provided here:

Solving a Pinhole Math Problem

1. Describe the scenario in words
2. Make a sketch
3. Review what you know about this phenomenon (the relevant central ideas)
4. Draw a careful ray diagram. Include:
   • light source
   • object and projection
   • eye
   • 3 example rays from light source (from top, middle, bottom of object) that pass through the pinhole to form the projection
   • light rays traveling to eye
   • gap representing pinhole (or small circle)
   • arrows to show direction light rays are traveling
5. Tell the ‘story’ of the ray diagram with the relevant central ideas to explain why the projection is upside down.  Describe what happens to each example ray separately.
6. Label angles and sides of triangles. Define symbols with words and record any numbers you know or can estimate.
7. Justify that the triangles are similar.
8. Write an equation that relates the sides of the triangles using words to describe the quantities.
9. Rewrite your equation using your symbols.
10. Algebraically isolate the unknown quantity.
11. Plug-in numbers and calculate answer.
12. Check answer. Does the number you get from the calculation seem reasonable? Explain.

3. Using pinhole phenomena to estimate the Sun’s diameter with friends and/or family members

Question 1.17 What happens when estimating the diameter of the Sun with friends or family members?

A student reflected upon engaging her sister and mother in estimating the diameter of the Sun as follows:

To explore pinhole phenomena, I involved my younger sister to perform the same experiment we did in class. I first explained what we did and she stated that she has never heard of it before. Because she said she has never heard of pinhole phenomena, I was excited to show her. I used the same materials and made my own pinhole on a sheet of tinfoil. My mom also helped by holding the meter stick. My mom also said she has never heard of it. My mom is a third-grade teacher so she was excited to try it out.

During the experiment, I asked them how big they thought the projection on the white sheet was. Like I did, they thought it looked like about one centimeter wide in diameter. My sister asked, “How can someone use such a small reflection of the sun to help find the diameter of the actual sun since the sun is so large and this is so small?” Because my mom and sister don’t have as much of a math background as I do, I was very careful about what questions I asked.

I started out by giving them a hint and asked, “Have you heard of similar triangles and corresponding angles?” I could tell my mom was a little lost because math isn’t her strong suit. Much like my sister, she is a lot better at literature. My sister said yes! I told them the distance from the sun is 100,000,000 miles, so by using the three dimensions, I asked, “How can we use equal fractions to find the missing dimension (diameter of sun)?”

My sister yelled, “Ratios!” My mom seemed a little lost so I then drew similar triangles a lot like we did in class and labeled each dimension we knew. I explained how the sides and angles were proportionate, which is how we could create equal ratios. I asked them if our units needed to be the same and my sister said yes. As a group, we found the same results as we did in class.

My sister took calculus in high school and my mom doesn’t know much about math. Having those two different types of “students” that were at either end of the spectrum, gave me the opportunity to practice for when I am trying to explain to two students where one is at a higher level than the other. I wanted my sister to explain the concept to my mom because I know that some students learn better when they explain things to other students.

Through this experience, I learned that when you have two students where one understands the concept faster than the other, it’s important to let both students talk but also let the one student who understands, explain what they know to the other student. This helps them learn from each other.

I also learned to make sure I give time for the student that doesn’t understand to ask any questions they have and not let the other student do all the work. I know it’s important to make sure you leave a lesson knowing that both students understand the process.

The last thing I learned was how to ask questions to give a hint when the student is completely lost without giving them the answer. I understand how frustrating it is when the teacher asked questions you have no idea what the answer is. It’s sometimes better to give them a nudge when they are completely lost in the beginning and let them do the rest of the work once they understand how they are going to find the outcome.

Physics student, Fall 2016

4. Some thoughts about the nature of science in this context:

This completes an example of the process outlined for each unit:

Students first identify resources by reflecting upon what they already know about a topic, such as the nature of light.

Next, they develop some central ideas based on evidence, such as light leaves a point on most sources in many directions and light can be envisioned as rays traveling in straight lines.

They can use those ideas to explain an interesting phenomenon, such as why the projection of a light bulb seen through a pinhole camera is upside down.

Then they develop mathematical representations of the phenomenon, such as an equation stating that the ratio of the height of the light source to the distance of the source from the pinhole is equal to the ratio of the height of the projection to the distance of the projection from the pinhole.

Finally, they use those mathematical representations to estimate an interesting quantity, such as the diameter of the Sun. It is not possible for anyone to travel to the Sun and use a measuring device to directly measure its diameter. An estimate is feasible, however, using simple equipment, some additional information from a reliable source, and the scientific process developed here.

This process illustrates the strength of scientific ways of knowing. One may be able to estimate a quantity of interest through a similar process of identifying resources, developing central ideas based on evidence, using these ideas to explain a puzzling phenomenon, figuring out ways to represent the phenomenon mathematically, and using those mathematical tools to estimate the quantity one wants to know.

Several students recognized that this estimation process might be useful in other contexts such as answering the question: ‘how big is the Moon”?  Note that making the decision to go there, or not, or to fund creating a settlement on the Moon, or not, is a question of a different kind, one that involves cultural issues and societal values as well as the technical and scientific knowledge needed.

Many students also experienced some of the human aspects of science in their surprise that the projection was upside down, frustration in making sense of the mathematics, and perhaps pleasure in persisting through to understanding what seemed for many to be an initially confusing experience.

The process involved in estimating the diameter of the Sun assumes that the ratio of small distances here on Earth can be compared to the ratio of very large distances in the solar system. This is an example of an aspect of the nature of science articulated in the US Next Generation Science Standards that scientific knowledge assumes an order and consistency in natural systems. Third to fifth grade students, for example, should understand that basic laws of nature are the same everywhere in the universe (NGSS, Lead States, 2013,  Appendix H) https://www.nextgenscience.org/resources/ngss-appendices).