VIII. Using Mathematical Representations to Estimate a Quantity of Interest

Qualitative approaches, such as interpreting the shapes of graphs, were helpful in gaining a conceptual understanding about what is happening when mixing hot and cold water. Quantitative approaches make possible the making of predictions and estimation of quantities that may be of interest.

A. Solving a thermal math problem

In this section, we demonstrate how to solve thermal math problems in the context of mixing hot and cold water. We assume that the specific heat of water is the same over the range of temperatures between freezing at 0ºC and boiling at 100ºC. We also assume that the specific heat of water is the same as the specific heat of fluids such as tea, milk, and cocoa when working thermal energy problems involving mixing these substances. The same mathematical process also applies in more complex situations in which the specific heat of the hot material differs, however, from the specific heat of the cold material, when, for example, a piece of hot metal is submerged into a cooling bath.

Question 2.14 How can one use mathematical representations of thermal phenomena to estimate a quantity of interest?

After developing both experimental and theoretical ways to describe what happens when mixing hot and cold water, one can use that knowledge to generate and solve thermal math problems.

To make up a thermal math problem, decide on a scenario and specify three of the four variables involved if only considering changes in temperature:  mass of hot water, mass of cold water, magnitude in change in temperature of hot water, or magnitude of change in temperature of cold water. Also include information about one of the initial temperatures or equilibrium temperatures. Use mass units (grams, kilograms) or parts rather than volume units. If the scenario involves materials with difference specific heats, be sure to include that information in stating the problem and in calculating the answer.

As with solving pinhole math problems, the goal in solving a thermal math problem is not the “answer.” The goal is to build your ability to help  someone else understand what to do and why. Start by helping the learner to understand what is happening by describing the scenario verbally with words and visually with a sketch. Next review the physics involved by stating what the relevant central ideas are. Also draw a qualitative graph and use it and the central ideas to explain what is happening. Then describe the graph mathematically, being clear about what each symbol represents. Justify the equation that relates the quantities represented; write the equation in both words and symbols. Finally, solve for the unknown in symbols before substituting values. After calculating an answer, be sure to also discuss why that answer seems reasonable.

In facilitating a conversation with someone about thermal phenomena, ask questions rather than tell answers throughout this process. In solving a thermal math problem for homework, follow the format provided here:

Format for Solving a Thermal Math Problem

1. State the problem in words
2. Make a sketch of the amounts to be mixed
3. Review what you know about the physics of this phenomenon: summarize the conceptual model by stating the relevant central ideas
4. Draw a graph representing the problem: use a ruler to make straight perpendicular axes, draw flat horizontal lines to represent temperatures that are not changing before the two substances are mixed, add the shared equilibrium temperature after the mixing, check that you have drawn the appropriately shaped graph for mixing more hot than cold or more cold than hot or equal amounts. Draw the graph to indicate whether the changes in temperature happen very quickly or are gradual processes.
5. Tell the ‘story’ of the graph with the relevant central ideas to explain why the equilibrium temperature is likely to be where you have drawn it (nearer the temperature of the initial hot water, initial cold water, or in the middle.)
6. Represent this scenario mathematically: State the equation in words that relates the masses of the substances mixed and their changes in temperature. Use the experimental form of the equation derived from your exploration, including specific heats if the materials differ, or the theoretical form based on the Conservation of Energy.  Justify the use of the equal sign accordingly.
7. Define symbols, state equation in symbols, and express how you are envisioning this equation.
8. Solve for the unknown in symbols
9. Record given values and estimate any needed
10. Substitute values and calculate answer
11. Check answer: why does the number you get from the calculation seem reasonable?

• Complete the process of generating and solving a thermal math problem before looking at a slightly modified example of student work.

1. Example of student work generating and solving a thermal math problem

            The problem stated in words is: If you have 180 grams of hot tea, and you want to cool it down by 20 degrees Celsius by adding 60 grams of cold water, how much will the temperature of the cold-water change? Assume all of the energy lost by the tea is gained by the cold water and that the specific heats of tea and water are the same. The initial temperature of the cold water is 15 degrees Celsius. After finding the change in temperature of the cold water, find the equilibrium temperature and the initial temperature of the hot tea.

            (As shown in Fig. 2.20), below is a sketch of the situation.

(As shown in Fig 2.21), below is a graph that represents the problem. The first relevant idea is, when mixing unequal amounts of hot and cold water, the ratio of the amount of hot water to the amount of cold water is approximately equal to minus the ratio of the change in temperature of the cold water to the change in temperature of the hot water. Next, the energy lost by the hot object equals the product of its mass, specific heat, and change in temperature. Finally, energy is conserved: energy lost = energy gained, so when mixing hot and cold water, the (magnitude of the) energy lost by the hot water equals (the magnitude of) the energy gained by the cold water.

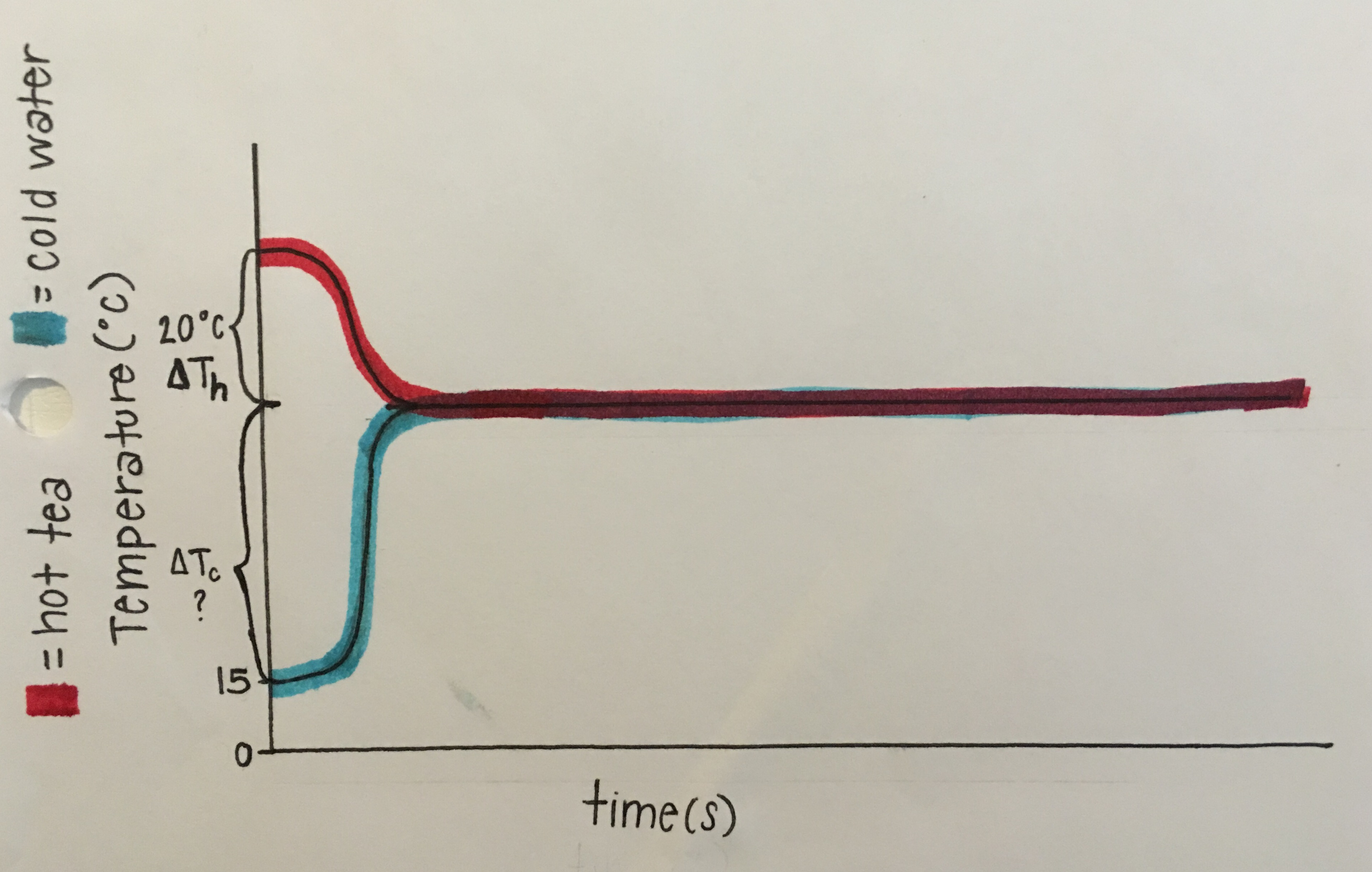
            Symbols that are defined for the relevant quantities are m_h= mass of hot tea, m_c= mass of cold water, ∆T_c= change in Temperature of cold water, and ∆T_h= change in Temperature of hot tea. An algebraic equation that relates these quantities in symbols is

[latex]\frac{\text{m}_h}{\text{m}_c} \approx \frac{-\Delta T_c}{\Delta T_h} [/latex]

The same equation in words is  

[latex]\frac{\text{Mass of hot tea}}{\text{Mass of cold water}} \approx \frac{-\text{change in Temperature of cold water}}{\text{change in Temperature of hot tea}}[/latex]

This equation is justified because it is equivalent to the theoretical statement of the Law of Conservation of Energy – (m_h c_w ∆T_h ) = ( m_c c_w ∆T_c), assuming that no energy is gained by the environment such as the cup and air.

The unknown and the quantity to be calculated is the change in temperature of the cold water, ∆T_c. The symbols for the quantities I have provided in the problem statement and their numerical values are m_h= 180 grams, m_c= 60 grams, and ∆T_h= – 20°C.

The equation solved algebraically for the unknown is  [latex]{\Delta T_c} = -{\Delta T_h} \frac{{(\text{m}_h)}}{(\text{m}_c)}[/latex].

When I substitute the values above for the known quantities and then calculate the answer I get

[latex]{\Delta T_c} = -({-20^{\circ}C}) \frac{\text{(180 grams)}}{\text{(60 grams)}} = {\text60}^{\circ}C[/latex]

This answer is reasonable because there was a lot more hot water than cold water which means that the temperature of the mixture will be closer to the initial temperature of the hot water than the initial temperature of the cold water. This means that the cold water should have a greater temperature change than the hot water. The hot water had a temperature change of -20°C, so this answer of the cold water having a temperature change of 60°C is reasonable.

The initial temperature of the cold water was 15°C. Since we know the initial temperature of the cold water, we are able to use equations to solve for the initial temperature of the hot water and for the equilibrium temperature. I can now write an algebraic equation for the unknown of the equilibrium temperature, T_e. That equation is T_e  =  T_c   + ∆T_c. When I substitute the values in I get T_e = 15°C + 60°C= 75°C.

Then, I can write an algebraic equation for the unknown of the initial temperature of the hot tea. That equation is ∆T_h. = T_e  – T_h   so T_h  = T_e  – ∆T_h  When I substitute the values in I get T_h = 75°C – (-) 20°C= 95°C. This initial hot temperature is 95°C which is below boiling point and the initial cold temperature is 15°C which is above freezing, which both seem reasonable. Cooling tea down to 75°C also seems reasonable because this is below boiling point but above freezing. If a student spills the tea, it is not hot enough to burn them.

Physics Student, Spring 2016

Actually 75°C is hot enough to burn one severely. Recommendations are for ‘hot’ liquids to be at 60°C or less. https://www.ncbi.nlm.nih.gov/pubmed/18226454