Unit 2: Exploring the Nature of Thermal Phenomena
VII. Developing a Mathematical Representation of Thermal Phenomena Based on Theoretical Considerations
The experimental approach discussed above focused only upon the relative masses of the hot and cold water and their relative changes in temperature. No mention was made of the energy flowing from the hot to the cold water. An earlier section in this unit (II.B, Table II.1, page 11) had developed the following central idea:
A temperature difference implies a flow of energy from hotter objects to colder objects.
In this section, we consider how to express theoretical ways of thinking about that transfer of energy from the hot water into the cold water. This involves use of the Law of Conservation of Energy, that the total energy in a closed system does not change, that energy lost by the hot water is gained by the cold water and its surroundings.
A. Considering what happens when energy flows from hot water into cold
Question 2.11 What theoretical considerations can provide insights into what is happening when energy flows from the hot water into the cold water?
Energy is measured in different units in different contexts. (see https://www.aps.org/policy/reports/popa-reports/energy/units.cfm . In this course, we use the energy unit of a calorie.
A calorie is the amount of energy needed to change the temperature of one gram of water by one degree Celsius at standard atmosphere pressure and 20°C. This amount of energy is very small; the calories typically discussed in the context of food are kilocalories, 1000 of the calories discussed here. A kilocalorie is the energy needed to change the temperature of one kilogram of water by one degree Celsius at standard atmospheric pressure and 20°C.
There are many different materials one could use in exploring thermal phenomena. The amount of energy needed to raise the temperature of one gram of a material by one degree Celsius is called the material’s specific heat.
Water is the standard. One calorie of energy is needed to raise a mass of one gram of water by one degree Celsius at standard atmospheric pressure and 20°C. The symbol for specific heat is c. In this course, water’s specific heat is written cw = 1.0 cal/(g°C).
Note that the units for mass and temperature both are in the denominator of this expression for specific heat. This is a mathematical way of stating that the change in energy for water is one calorie to change each gram by one Celsius degree. The dimensions of specific heat, c, are:
Dimensions of specific heat: [latex]\frac{\text{(energy)}}{\text{(mass)(temperature)}}[/latex]
The units are: [latex]\frac{\text{(calories)}}{\text{(grams)(degrees Celsius)}}[/latex]
To express mathematically how much energy is gained or lost when something is warmed or cooled:
- What might be the effect of how much “stuff” is involved?
- What might be the effect of the kind of “stuff” one has?
- What might be the effect of how much the temperature changes?
- How can you combine measures of these effects mathematically to estimate the energy lost by the hot water?
Complete Table II.3 and write a summary about expressing these theoretical considerations mathematically.
| Table II.3 Developing a mathematical expression for change in energy | |||
|---|---|---|---|
| Sketch | Mathematical representation | Theoretical consideration | Vocabulary |
| m | The bigger the mass of material, the more energy is lost or gained | Mass | |
| c | The energy lost or gained also depends upon a property of the material, how much energy is needed to change the temperature of a mass of one gram by one degree Celsius, its specific heat. | Specific heat | |
| ∆T | The bigger the temperature change, the more energy is lost or gained. | ∆T, delta T, represents “change in temperature”
∆T = Tf – Ti |
|
|
m c ∆T
|
The change in energy equals the product of mass, specific heat, and change in temperature. | Change in energy | |
After completing Table II.3, look at an example of student work and consider nuances about mathematical representations of thermal phenomena based upon theoretical considerations.
1. Example of student work about developing a mathematical expression for a change in energy
This section is an example of using mathematics to express some theoretical insights. The ratios above do not directly address what is happening conceptually in terms of energy transfers when mixing hot and cold water. The considerations that one must make, including how much material one has, what kind of material this is, and how much the temperature changes, are indicated in (the table shown in Fig. 2.19 below.)
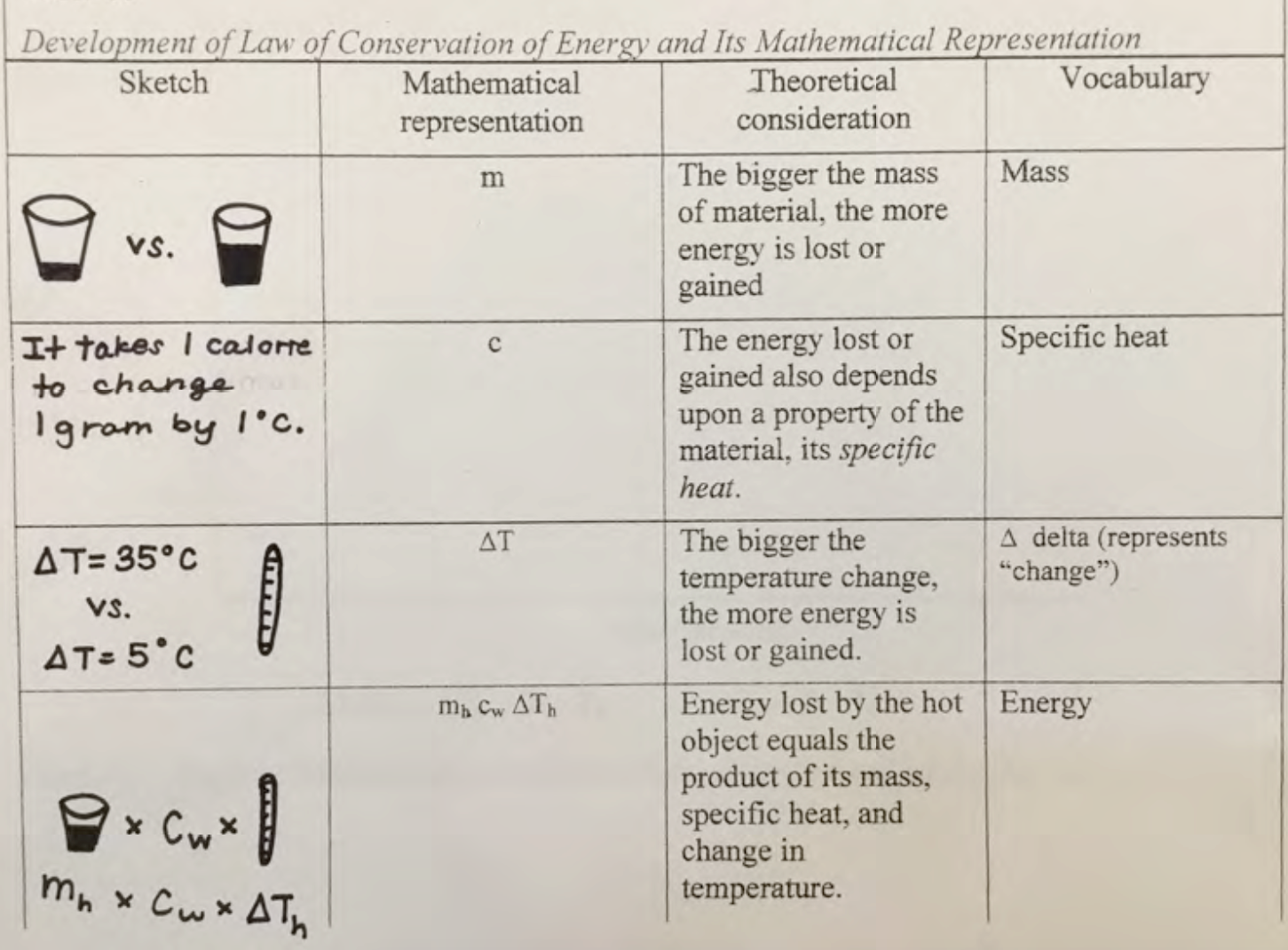
The bigger the mass of material, the more energy is lost or gained. The first row in (the table) presents this idea. For this experiment, the more water that there was, the more energy was lost or gained. This is because the more mass that a material has, the more energy it will take to change things about the material, such as its temperature. Since it takes 1 calorie to change 1 gram of water 1°C, the greater the mass of the material, the more calories were needed, which means that more energy was lost or gained.
The energy lost or gained also depends upon a property of the material, its specific heat. The second row in (the table) shows this idea. Specific heat is defined as, c = the amount of energy (lost or gained) to change the temperature of one gram of material by one degree Celsius. Water is the standard, cw = one calorie of energy that is lost or gained when the temperature of one gram of water changes by one degree Celsius (at standard atmospheric pressure and 20ºC).
The bigger the change in temperature, the more energy is lost or gained. This idea is displayed in the third row in (the table). Since it takes 1 calorie to change 1 gram of water 1°C, the greater the temperature change, the more calories were needed, meaning that more energy was lost or gained.
Energy lost by the hot object equals the product of its mass, specific heat, and change in temperature. This idea is presented in the fourth row of (the table). These variables are multiplied to express mathematically the energy lost by the hot water mhcw ∆Th, because in order to know how much energy is lost, you have to know how much material is losing energy, the specific heat of that material, and the change in temperature of that material. If you know all of that information, you are able to multiply the three values together in order to determine the amount of energy that is lost.
Physics student, spring 2016
2. An analogy to specific heat and the mathematical expression for change in energy
This progression of mathematical thinking can seem challenging. However, exploring both experimental and theoretical approaches to relating quantities is an important part of modeling phenomena in science.
The process of developing such mathematical expressions can increase comfort with using subscripts, in interpreting expressions involving multiple symbols, and in deepening understanding of what multiplication represents in viewing mhcw∆Th as a meaningful expression for how much a mass mh of hot water, with specific heat cw , changes in energy when it cools by ∆Th degrees in temperature when mixed with cold water.
Sometimes it helps to consider an analogous situation:
- How would you estimate the budget for a project if the basic cost, c, is the number of dollars paid for each hour worked by each person?
What would be the estimated budget for the project, for example, if you hired 5 people at a cost of $15/hour for each person who worked for 2 hours?
You would need: [latex]\text{(5 people)}(\frac{\text{\$ 15}}{\text{each person, each hour}})\text{(2 hours)} = \$ 150[/latex]
Note that multiplication is indicated here by parentheses ( ) rather than by x’s in order to avoid confusion with situations in which an x represents an unknown, which often occurs in algebraic equations.
In general, if n = the number of people, c = the cost for each person for each hour, and ∆t = the estimated duration (final clock reading – initial clock reading), i.e., the time in hours needed for this number of people to complete the project, the budget would be:
[latex]\text{Number of dollars needed} = \text{(number of people)}(\frac{\text{dollars}}{\text{person hour}})\text{(estimated number of hours)}[/latex]
Budget needed for the project = nc∆t
The mathematical reasoning for energy transfer is exactly the same:
- How would you calculate the change in energy if the mass of the material was m, the specific heat of a material is “c,” which is the number of calories needed for an energy change of one gram for each degree C change in temperature, and the material changed in temperature ∆T?
If m = mass in the number of grams,
c = energy in calories needed for each gram for each degree C change in temperature, and
ΔT = change in temperature in number of degrees C,
the change in energy would be:
change in energy = mcΔT
Although it may be hard to view mc∆T as a meaningful expression for change in energy, one can focus on dimensions and see this as:
[latex]\text{change in energy} = \frac{\text{(mass) (energy) (temperature)}}{\text{(mass)(temperature)}} = \text{energy}[/latex]
or focus on units and see this as:
[latex]\text{change in energy} = \frac{\text{(# of grams) (# of calories ) (# of degrees C change in T ) }}{\text{(gram)(degree C)}} = \text{# of calories}[/latex]
It can be helpful in working thermal problems, to step back and evaluate the dimensions on both sides of an equation to check that one has not made an algebraic mistake in manipulating an equation to solve for an unknown.
B. Considering the Law of Conservation of Energy
Question 2.12 How does the energy gained by the cold water compare to the energy lost by the hot water, assuming no energy is gained by the surrounding environment?
The total change in energy should equal the change in energy of the hot water plus the change in energy of the cold water plus any change in energy of the surroundings. This is a statement based on the Law of Conservation of Energy. The word “conservation” here means that the total amount of energy does not change when energy flows from one part of a system to another or from one form into another.
This means that the total change in energy when hot and cold water mix should equal 0. Total change in energy = change in energy of hot water + change in energy of cold water + any energy flowing into the surroundings.
If no energy flows into the surroundings:
mhcw∆Th + mccw∆Tc = 0 Law of Conservation of Energy
Note that because ∆Th = Thf – Thi and the final temperature is always less than the initial temperature for the hot water, ∆Th is always negative, and therefore mhcw∆Th is always negative, which can be interpreted as the energy lost by the hot water.
The energy lost by the hot water + energy gained by the cold water = 0, makes sense as long as none of the energy lost by the hot water flows into the air or the containers. This also means that the magnitude of the energy lost by the hot water equals the magnitude of the energy gained by the cold water as long as none of the energy lost by the hot water flows into the air or the containers.
Question 2.13 How are these experimental and theoretical approaches related?
According to the Law of Conservation of Energy, the total energy does not change: Total change in energy = change of energy for hot water + change in energy for cold water = 0 if no energy flows into the surroundings.
mhcw∆Th + mccw∆Tc = 0 Law of Conservation of Energy
This algebraic relationship also can be written as: mhcw∆Th = – mccw∆Tc
This equation tells us that the change in energy of the hot water is equal and opposite to the change in energy of the cold water
This equation can be simplified by dividing both sides by cw, mc and ∆Th in order to make ratios of m’s and ∆T’s:
[latex]\frac{m_h}{m_c} = - \frac{\Delta T_c}{\Delta T_h}[/latex]
This is the equation developed experimentally with approximate results! Thus the theoretical approach confirms the relationship evident in the patterns of data obtained by measuring temperature changes when mixing various configurations of hot and cold water.
One can work forwards from the experimental result by multiplying both sides by mc and ∆Th
and including the specific heat of water on both sides to obtain the theoretical result:
mh cw∆Th = – mccw∆Tc
One also can express this relationship in terms of magnitudes:
|mh cw∆Th| = |mccw∆Tc|
Any of these approaches can be used in estimating a thermal quantity of interest.

